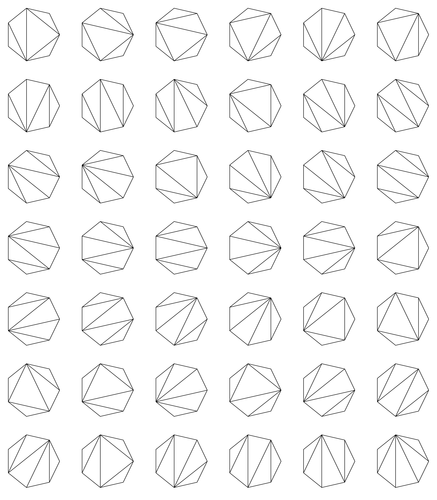
Example: Polygon division

Published 2008-10-09 | Author: Eric Détrez

This example shows the solution of Euler’s polygon division problem for a heptagon. The problem is to find in how many ways a plane convex polygon of n sides can be divided into triangles. The solution is given by the Catalan number. For a heptagon the number is 42.

Download as: [PDF] [TEX]

Do you have a question regarding this example, TikZ or LaTeX in general? Just ask in the LaTeX Forum.
Oder frag auf Deutsch auf TeXwelt.de. En français: TeXnique.fr.

\documentclass{minimal}
\usepackage{tikz}

\begin{document}
% Macro for drawing a heptagon   
\def\hepta{\draw(A) -- (B) -- (C) -- (D) -- (E) -- (F) -- (G) -- cycle;}

% Macro for drawing polygon diagonals. 
% Example \slice{A/C,C/E,E/G,C/G}

\newcommand{\slice}[1]{%
    \hepta
    \draw \foreach \x/\y in {#1} {(\x)--(\y)};

}


\begin{tikzpicture}
    % Define the heptagon coordinates
    \coordinate (A) at (-0.76,1.54);
    \coordinate (B) at (-0.76,0.69);
    \coordinate (C) at (-0.10,0.16);
    \coordinate (D) at (0.73,0.35);
    \coordinate (E) at (1.1,1.11);
    \coordinate (F) at (0.73,1.88);
    \coordinate (G) at (-0.10,2.07);


\matrix[column sep=0.8cm,row sep=0.5cm]
{
    \slice{A/C,C/E,E/G,C/G}&
    \slice{A/C,C/E,E/G,A/E}&

    \slice{A/C,C/E,A/E,A/F}&
    \slice{A/C,C/E,C/F,A/F}&
    \slice{A/C,C/E,C/F,C/G}&

    \slice{A/C,C/F,D/F,A/F}\\
    \slice{A/C,C/F,D/F,C/G}&
    \slice{A/C,C/G,D/G,D/F}&

    \slice{A/C,C/G,D/G,E/G}&
    \slice{A/C,A/D,D/F,A/F}&
    \slice{A/C,A/D,D/F,D/G}&

    \slice{A/C,A/D,D/G,E/G}\\
    \slice{A/C,A/D,A/E,E/G}&
    \slice{A/C,A/D,A/E,A/F}&

    \slice{A/D,B/D,D/F,A/F}&
    \slice{A/D,B/D,D/F,D/G}&
    \slice{A/D,B/D,D/G,E/G}&

    \slice{A/D,B/D,A/E,E/G}\\
    \slice{A/D,B/D,A/E,A/F}&
    \slice{A/E,B/E,B/D,E/G}&

    \slice{A/E,B/E,B/D,A/F}&
    \slice{A/E,B/E,C/E,E/G}&
    \slice{A/E,B/E,C/E,A/F}&

    \slice{A/F,B/F,B/D,D/F}\\
    \slice{A/F,B/F,B/D,B/E}&
    \slice{A/F,B/F,B/E,C/E}&

    \slice{A/F,B/F,C/F,C/E}&
    \slice{A/F,B/F,C/F,D/F}&
    \slice{B/G,B/D,D/F,B/F}&

    \slice{B/G,B/D,D/F,D/G}\\
    \slice{B/G,B/D,D/G,E/G}&
    \slice{B/G,B/D,B/E,E/G}&

    \slice{B/G,B/D,B/E,B/F}&
    \slice{B/G,B/E,C/E,E/G}&
    \slice{B/G,B/E,C/E,B/F}&

    \slice{B/G,B/F,C/F,C/E}\\
    \slice{B/G,B/F,C/F,D/F}&
    \slice{B/G,C/G,C/E,E/G}&

    \slice{B/G,C/G,C/E,C/F}&
    \slice{B/G,C/G,C/F,D/F}&
    \slice{B/G,C/G,D/G,D/F}&

    \slice{B/G,C/G,D/G,E/G}\\
};
\end{tikzpicture}

\end{document}